import numpy as np
import matplotlib.pyplot as plt
import odesolver as ode
%pylab inline
Populating the interactive namespace from numpy and matplotlib
1. First, build a Runge-Kutta implementation of the three-body equations, using the same type of decomposition/rewriting process that you used to get the two-body equations in the previous problemset. Use an adaptive timestep integrator.
Test your program using the initial conditions that you derived previously for two of the stars, and starting a third star, of equal mass and at rest, several thousand units away. The binary star should be the same if the third is far enough away.
Given the following system:
\[\begin{aligned} \ddot{\vec{r} }_1 &=& -G \left( m_2 \frac{\vec{r}_1 - \vec{r}_2}{ {|(\vec{r}_1 - \vec{r}_2)|}^3} + m_3 \frac{\vec{r}_1 - \vec{r}_3}{ {|(\vec{r}_1 - \vec{r}_3)|}^3} \right)\\ \ddot{\vec{r} }_2 &=& -G \left( m_1 \frac{\vec{r}_2 - \vec{r}_1}{ {|(\vec{r}_2 - \vec{r}_1)|}^3} + m_3 \frac{\vec{r}_2 - \vec{r}_3}{ {|(\vec{r}_2 - \vec{r}_3)|}^3} \right)\\ \ddot{\vec{r} }_3 &=& -G \left( m_1 \frac{\vec{r}_3 - \vec{r}_1}{ {|(\vec{r}_3 - \vec{r}_1)|}^3} + m_2 \frac{\vec{r}_3 - \vec{r}_2}{ {|(\vec{r}_3 - \vec{r}_2)|}^3} \right)\\ \end{aligned}\]| (Where in this case $ | \vec{r} | $ indicates the $\mathcal{L}_2$, or Euclidean norm as a measurement of distance.) |
We can rewrite to the following form.
\[\begin{aligned} \dot{x}_1 &=& u_1\\ \dot{y}_1 &=& v_1\\ \dot{z}_1 &=& w_1\\ \ddot{x}_1 &=& -G \left( m_2 \frac{x_1 - x_2}{ {\left[{(x_1 - x_2)}^2 + {(y_1 - y_2)}^2 + {(z_1 - z_2)}^2\right]}^{(3/2)} } + m_3 \frac{x_1 - x_3}{ {\left[{(x_1 - x_3)}^2 + {(y_1 - y_3)}^2 + {(z_1 - z_3)}^2\right]}^{(3/2)} } \right)\\ \ddot{y}_1 &=& -G \left( m_2 \frac{y_1 - y_2}{ {\left[{(x_1 - x_2)}^2 + {(y_1 - y_2)}^2 + {(z_1 - z_2)}^2\right]}^{(3/2)} } + m_3 \frac{y_1 - y_3}{ {\left[{(x_1 - x_3)}^2 + {(y_1 - y_3)}^2 + {(z_1 - z_3)}^2\right]}^{(3/2)} } \right)\\ \ddot{z}_1 &=& -G \left( m_2 \frac{z_1 - z_2}{ {\left[{(x_1 - x_2)}^2 + {(y_1 - y_2)}^2 + {(z_1 - z_2)}^2\right]}^{(3/2)} } + m_3 \frac{z_1 - z_3}{ {\left[{(x_1 - x_3)}^2 + {(y_1 - y_3)}^2 + {(z_1 - z_3)}^2\right]}^{(3/2)} } \right)\\ \dot{x}_2 &=& u_2\\ \dot{y}_2 &=& v_2\\ \dot{z}_2 &=& w_2\\ \ddot{x}_2 &=& -G \left( m_1 \frac{x_2 - x_1}{ {\left[{(x_2 - x_1)}^2 + {(y_2 - y_1)}^2 + {(z_2 - z_1)}^2\right]}^{(3/2)} } + m_3 \frac{x_2 - x_3}{ {\left[{(x_2 - x_3)}^2 + {(y_2 - y_3)}^2 + {(z_2 - z_3)}^2\right]}^{(3/2)} } \right)\\ \ddot{y}_2 &=& -G \left( m_1 \frac{y_2 - y_1}{ {\left[{(x_2 - x_1)}^2 + {(y_2 - y_1)}^2 + {(z_2 - z_1)}^2\right]}^{(3/2)} } + m_3 \frac{y_2 - y_3}{ {\left[{(x_2 - x_3)}^2 + {(y_2 - y_3)}^2 + {(z_2 - z_3)}^2\right]}^{(3/2)} } \right)\\ \ddot{z}_2 &=& -G \left( m_1 \frac{z_2 - z_1}{ {\left[{(x_2 - x_1)}^2 + {(y_2 - y_1)}^2 + {(z_2 - z_1)}^2\right]}^{(3/2)} } + m_3 \frac{z_2 - z_3}{ {\left[{(x_2 - x_3)}^2 + {(y_2 - y_3)}^2 + {(z_2 - z_3)}^2\right]}^{(3/2)} } \right)\\ \dot{x}_3 &=& u_3\\ \dot{y}_3 &=& v_3\\ \dot{z}_3 &=& w_3\\ \ddot{x}_3 &=& -G \left( m_1 \frac{x_3 - x_1}{ {\left[{(x_3 - x_1)}^2 + {(y_3 - y_1)}^2 + {(z_3 - z_1)}^2\right]}^{(3/2)} } + m_2 \frac{x_3 - x_2}{ {\left[{(x_3 - x_2)}^2 + {(y_3 - y_2)}^2 + {(z_3 - z_2)}^2\right]}^{(3/2)} } \right)\\ \ddot{y}_3 &=& -G \left( m_1 \frac{y_3 - y_1}{ {\left[{(x_3 - x_1)}^2 + {(y_3 - y_1)}^2 + {(z_3 - z_1)}^2\right]}^{(3/2)} } + m_2 \frac{y_3 - y_2}{ {\left[{(x_3 - x_2)}^2 + {(y_3 - y_2)}^2 + {(z_3 - z_2)}^2\right]}^{(3/2)} } \right)\\ \ddot{z}_3 &=& -G \left( m_1 \frac{z_3 - z_1}{ {\left[{(x_3 - x_1)}^2 + {(y_3 - y_1)}^2 + {(z_3 - z_1)}^2\right]}^{(3/2)} } + m_2 \frac{z_3 - z_2}{ {\left[{(x_3 - x_2)}^2 + {(y_3 - y_2)}^2 + {(z_3 - z_2)}^2\right]}^{(3/2)} } \right)\\ \end{aligned}\]We can express this with code.
def gen_orbit(g=1, m1=0.5, m2=0.5, m3=0.5):
def orbit(t, y):
"""
Three body problem.
y -> x1, y1, z1,
u1, v1, w1,
x2, y2, z2,
u2, v2, w2,
x3, y3, z3,
u3, v3, w3
"""
# All position vectors
positions = np.array([y[:3], y[6:9], y[12:15]])
# All velocity vectors
velocities = np.array([y[3:6], y[9:12], y[15:18]])
# Distances => [0 to 1, 1 to 2, 2 to 0]
distances = np.array([np.linalg.norm(positions[i] - positions[(i + 1) % 3])
for i in range(3)])**3
return np.array([
velocities[0][0],
velocities[0][1],
velocities[0][2],
-g * (m2 * ((positions[0][0] - positions[1][0]) / distances[0]) +
m3 * ((positions[0][0] - positions[2][0]) / distances[2])),
-g * (m2 * ((positions[0][1] - positions[1][1]) / distances[0]) +
m3 * ((positions[0][1] - positions[2][1]) / distances[2])),
-g * (m2 * ((positions[0][2] - positions[1][2]) / distances[0]) +
m3 * ((positions[0][2] - positions[2][2]) / distances[2])),
velocities[1][0],
velocities[1][1],
velocities[1][2],
-g * (m1 * ((positions[1][0] - positions[0][0]) / distances[0]) +
m3 * ((positions[1][0] - positions[2][0]) / distances[1])),
-g * (m1 * ((positions[1][1] - positions[0][1]) / distances[0]) +
m3 * ((positions[1][1] - positions[2][1]) / distances[1])),
-g * (m1 * ((positions[1][2] - positions[0][2]) / distances[0]) +
m3 * ((positions[1][2] - positions[2][2]) / distances[1])),
velocities[2][0],
velocities[2][1],
velocities[2][2],
-g * (m1 * ((positions[2][0] - positions[0][0]) / distances[2]) +
m2 * ((positions[2][0] - positions[1][0]) / distances[1])),
-g * (m1 * ((positions[2][1] - positions[0][1]) / distances[2]) +
m2 * ((positions[2][1] - positions[1][1]) / distances[1])),
-g * (m1 * ((positions[2][2] - positions[0][2]) / distances[2]) +
m2 * ((positions[2][2] - positions[1][2]) / distances[1]))
])
return orbit
def midpoint(points):
""" Returns midpoint between arbitrary number of points """
return np.sum(points, axis=0) / len(points)
def plotdata(data, npoints):
plt.style.use('ggplot')
fig = plt.figure(figsize=(12, 8))
ax = fig.add_axes([0.1, 0.1, 0.8, 0.8])
for i in range(npoints):
# Plot each mass trajectory
ax.plot(data[:, i * 6], data[:, (i * 6) + 1], label=r'$m{}$'.format(i))
# Plot Mass labels and lines
ax.annotate(r'$m{}$'.format(i),
xy=(data[-1, i * 6], data[-1, (i * 6) + 1]), xycoords='data',
xytext=(data[-100, i * 6], data[-100, (i * 6) + 1]), textcoords='data',
size=10,
arrowprops=dict(arrowstyle='simple',
fc='black',
connectionstyle='arc3'))
ax.scatter([data[-1, i * 6] for i in range(npoints)],
[data[-1, (i * 6) + 1] for i in range(npoints)], s=50)
# Plot midpoint trajectory
centerline = midpoint([data[:, (i * 6):((i * 6) + 3)] for i in range(npoints)])
ax.plot(centerline[:, 0], centerline[:, 1], 'k-', label='Center of Mass')
ax.scatter(centerline[-1, 0], centerline[-1, 1], marker='x')
# Legend
plt.legend(bbox_to_anchor=(0., 1.02, 1., .102), loc=3,
ncol=4, mode="expand", borderaxespad=0.)
plt.show()
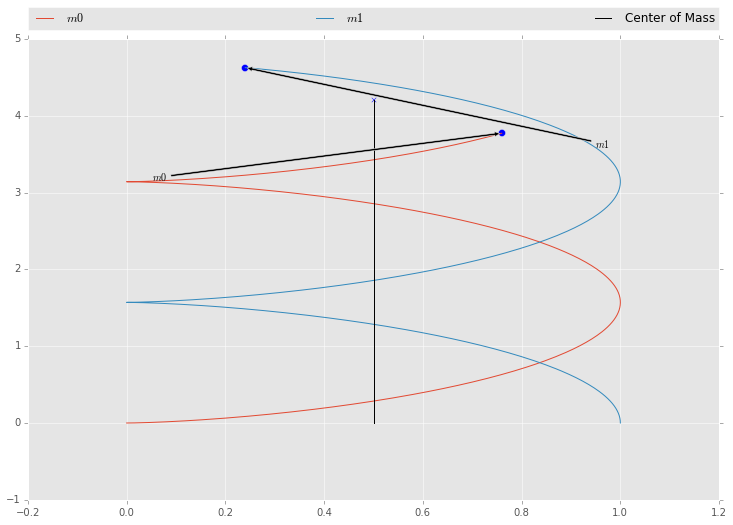
We can test this with our previous problem and note that the system is the same.
initial_state = np.array([
0, 0, 0, # m1 position
0, 0, 0, # m1 velocity
1, 0, 0, # m2 position
0, 1, 0, # m2 velocity
0, 1e9, 0, # m3 position
0, 0, 0 # m3 velocity
], dtype=np.float64)
data = ode.ark4(gen_orbit(), 0, initial_state, 0.001, 500, error=1e-10)
plotdata(data, 2)
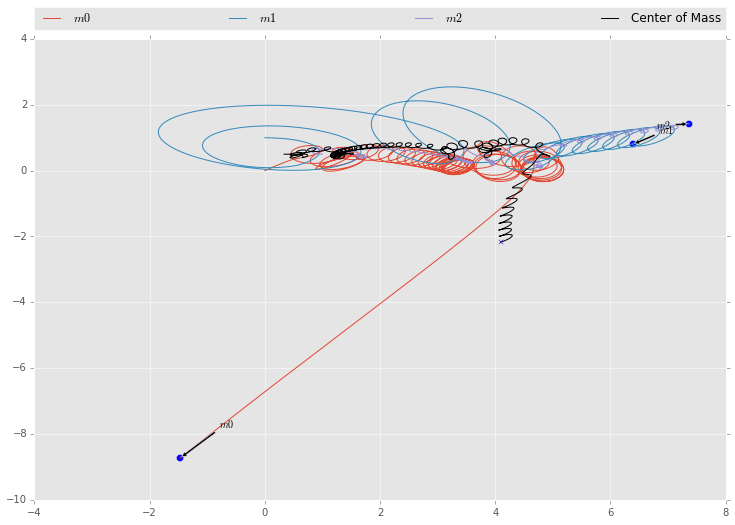
2. In the provided picture, the star whose mass is equal to that of each of the other two stars, sails into the binary from the right, along its centerline.
Start the binary at the initial conditions you derived previously and the other star 20 units away on the positive $y$ axis, moving directly towards the binary at $0.15$ units per second.
Run an integration of the three-body equations with these initial conditions. Plot the results in physical space. Does this look like the picture? Why or why not?
initial_state = np.array([
0, 0, 0, # m1 position
0, 0, 0, # m1 velocity
0, 1, 0, # m2 position
1, 0, 0, # m2 velocity
20, 0.5, 0, # m3 position
-0.15, 0, 0 # m3 velocity
], dtype=np.float64)
data = ode.ark4(gen_orbit(), 0, initial_state, 1e-4, int(2e3), error=1e-5)
plotdata(data, 3)
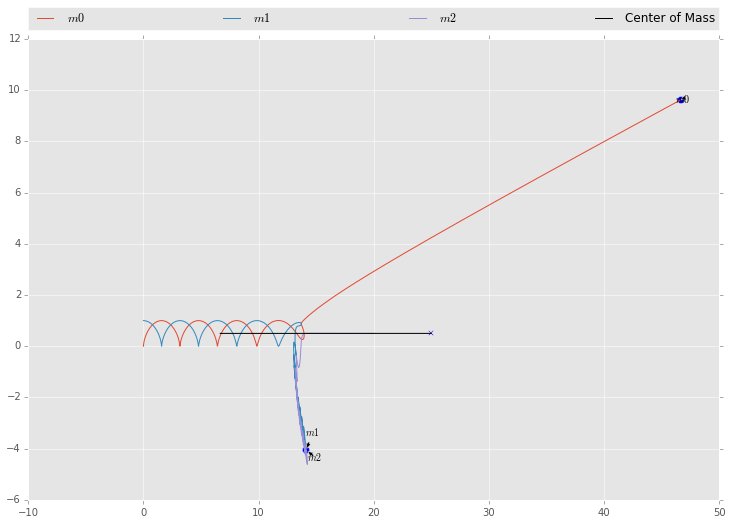
3. Play with the true anomaly, and describe the interactions you see. Ideally you’ll duplicate the picture, but other stuff can happen too.
initial_state = np.array([
0, 0, 0, # m1 position
0, 0, 0, # m1 velocity
0, 1, 0, # m2 position
1, 0, 0, # m2 velocity
15, 0.5, 0, # m3 position
-0.15, 0, 0 # m3 velocity
], dtype=np.float64)
data = ode.ark4(gen_orbit(), 0, initial_state, 1e-4, int(1e4), error=1e-5)
plotdata(data, 3)
initial_state = np.array([
0, 0, 0, # m1 position
0, 0, 0, # m1 velocity
0, 1, 0, # m2 position
1, 0, 0, # m2 velocity
20, 0.5, 0, # m3 position
-0.1, 0, 0 # m3 velocity
], dtype=np.float64)
data = ode.ark4(gen_orbit(), 0, initial_state, 1e-4, int(2e3), error=1e-5)
plotdata(data, 3)
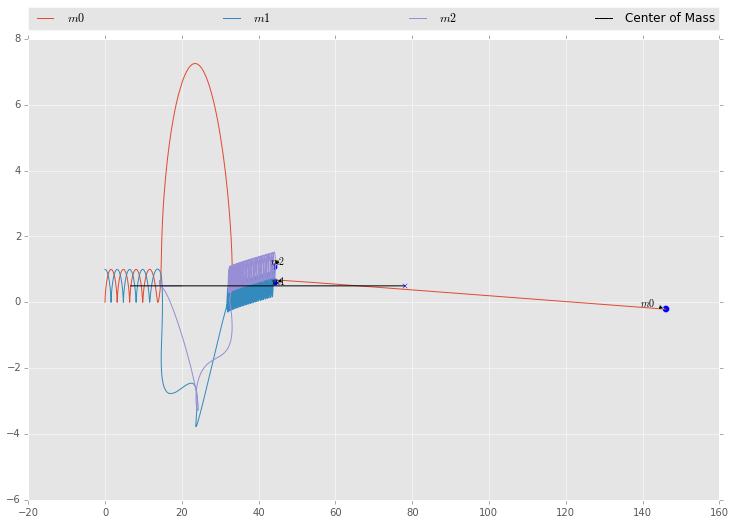
initial_state = np.array([
0, 0, 0, # m1 position
0, 0, 0, # m1 velocity
0, 1, 0, # m2 position
1, 0, 0, # m2 velocity
1, 0.5, 0, # m3 position
-0.15, 0, 0 # m3 velocity
], dtype=np.float64)
data = ode.ark4(gen_orbit(), 0, initial_state, 1e-4, int(1e4), error=1e-5)
plotdata(data, 3)
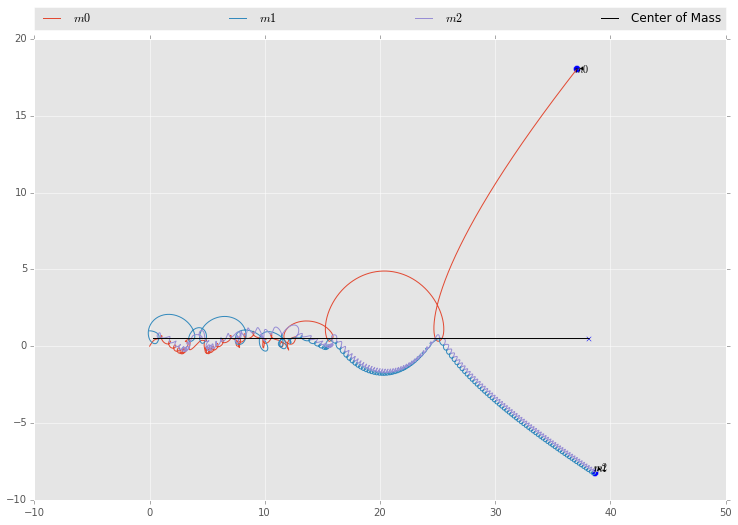
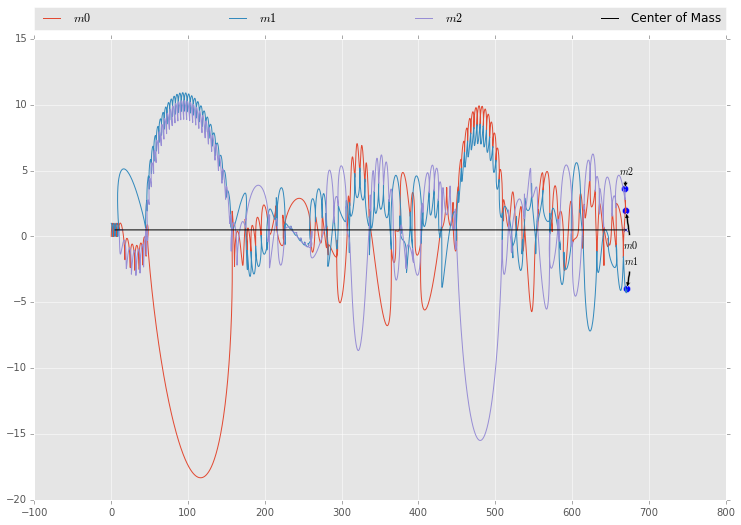
Now let’s play around with changing the gravity and mass of the particles.
initial_state = np.array([
0, 0, 0, # m1 position
0, 0, 0, # m1 velocity
0, 1, 0, # m2 position
1, 0, 0, # m2 velocity
1, 0.5, 0, # m3 position
-0.15, 0, 0 # m3 velocity
], dtype=np.float64)
data = ode.ark4(gen_orbit(g=1, m1=0.5, m2=0.5, m3=1),
0, initial_state, 1e-4, int(3e3), error=1e-5)
plotdata(data, 3)
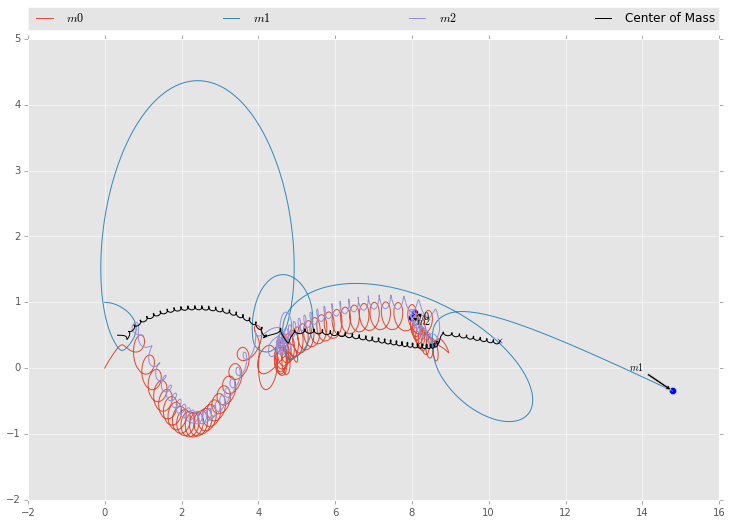
initial_state = np.array([
0, 0, 0, # m1 position
0, 0, 0, # m1 velocity
0, 1, 0, # m2 position
1, 0, 0, # m2 velocity
1, 0.5, 0, # m3 position
-0.15, 0, 0 # m3 velocity
], dtype=np.float64)
data = ode.ark4(gen_orbit(g=1, m1=0.1, m2=0.25, m3=1),
0, initial_state, 1e-4, int(3e3), error=1e-5)
plotdata(data, 3)